Background and history

Definition 1 Let be a first order system of evolution equations,

a ut = A (u)Dau + B (u)u,

where $u = u(x,t)$ indicates a “vector” function of dimension $s$ in $n+1 ℜ$ , $ut$ its time derivative, $a A (u)$ , a $s × s$ matrix valued vector, and $B (u)$ a $s × s$ matrix valued vector, whose components depend smoothly on $u$ , and $Da$ a partial derivative operator on $ℜn$ . The system is called symmetric hyperbolic at a solution $u0$ if there exists a neighborhood of $u0$ and a smooth, positive definite, symmetric matrix $H (u )$ on it such that:

H (u)Aa (u ) − Aa ⋆(u)H (u) = 0.

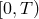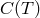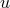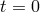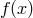This condition ensures that the Cauchy problem is well posed, namely that there exists a time interval $[0,T )$ and a constant $C (T)$ such that if initial data for $u$ is given at $t = 0$ , $u (x, 0) = f(x)$ , with $f(x)$ close enough to 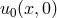$u0(x,0)$ in a certain norm, then for the same norm we have:

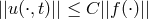
A simple example of a symmetric hyperbolic system is the wave equation, see Example I 2.2

Well posedness of the Cauchy problem for Einstein equations was established in the early fifties by Choquet-Bruhat [13 ], when the theory of evolutionary partial differential equations matured and general proofs for quasi-linear hyperbolic systems became available. With further refinement of the general theory, it was possible in the early seventies to improve on the result by lowering the minimal differentiability required in the proof, thus allowing more general initial data sets [39

], see also [17

] and [15 , 24 , 25 ]. These works used the harmonic gauge introduced by Lanczos in the twenties. For a discussion on the harmonic gauge see Section 3.1

In the seventies, with a new tool (the Weighted Sobolev Spaces) it became possible to enlarge the development region to contain asymptotically boosted slices relative to the initial slice, if the initial data were in a specific Weighted Sobolev space [21 , 16 ]. This result had two interesting by-products:

Even more, it was possible to use as the function spaces for the initial data the same weighed spaces in which the constraint equations were solved, thus, for the first time obtaining a global (in space) control on Einstein’s equations.

All the power of the techniques used in the above mentioned body of work was not enough to get a result which most people suspected would hold: the existence of complete, asymptotically flat space-times for generic, although small, initial data. An idea which made a breakthrough on this problem was to regularize Einstein’s equations in terms of a conformally rescaled metric on the corresponding conformally compactified space-time. Future and Null infinity are then at a finite distance, so standard, local in time, existence theorems can be used. Pursuing these ideas, Friedrich [27

, 26

] was able to craft the full, and therefore non-linear, Einstein equations for the conformal fields into a regular symmetric hyperbolic system, and so to create a formidable tool to study these problems. Earlier results using regularized equations for the conformal metric included the local linear stability of null infinity [35 ]¹ .

The variables used range from frames to Weyl tensor frame components. The regularization of the full equations is complicated and requires appending to the original Einstein equations the Bianchi identities as a new and independent set of variables. For the first time, the harmonic gauge was not used. This was also the first time a symmetric system was obtained which was not the mere and standard translation of quasi-linear second order wave equations into a first order system. This tool made it possible to show that, given any smooth and small enough initial data on a hypersurface reaching future null infinity at some cross section, a future development existed along which null infinity, to the future of such a hypersurface and up to future infinity, was included. A limitation of this technique is that initial data are not regular enough at space-like infinity to make the estimates work there, and so a complete, asymptotically flat space-time cannot be obtained, nor even a piece of null infinity starting from generic, although small, initial data on a space-like hypersurface; nevertheless see [22 ].

A different path was followed by Christodoulou and Klainerman [20

]. They also made use of the detailed structure of Einstein equations, but in physical space-time, to show global existence. The special structure of Einstein equations allows the use of other energy estimates beyond the traditional one. The estimates are boosted energies [45 , 46 , 44 ] and are crucial to establishing this global result. Christodoulou and Klainerman did not use a conformal compactification of space-time. They were able to obtain complete asymptotically flat space-times, i.e. asymptotically including space-like regions, out of rather generic initial data in a Cauchy surface reaching that infinity. Christodoulou and Klainerman found that at null infinity the differential structure does not seems to be $C ∞$ . 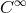That is, they claimed there are smooth initial data sets whose development is not smooth at null infinity and that only finite differentiability remains. Christodoulou and Klainerman also did not use a harmonic gauge condition. Rather, their strategy was to use the equations for tensorial quantities built out of higher order derivatives of the metric. After obtaining estimates for these tensorial quantities, estimates for the metric and its first derivatives were obtained from elliptic theory, and the maximal slicing condition.

As expected, both methods used detailed properties of the Einstein equations to assert global existence of small data solutions. It is believed that both methods use the same properties, but with different techniques. That is, the property that allows the conformal Einstein equations to be regular should be the same property that allows boosted energies to be estimated. In fact both make estimates in terms of the Bel–Robinson tensor. However, to my knowledge, this has not yet been fully explored.

1.1 Background and history