3 Modern Revival of the Argument
Discussion of the hole argument lay dormant for sixty years. Its modern revival came about as the result of debates about the reason for the delay of over two years between Einstein’s adoption of the metric tensor in 1913 and his formulation of the generally-covariant field equations for the metric at the end of 1915 (see Section 2).
3.1 Did Einstein misunderstand coordinate transformations?
The answer to this question hinges on the answer given to the question of why Einstein formulated the hole argument and held to it during this entire period. In 1982, Pais summarized the generally-accepted view:
In 1914 not only did he [Einstein] have some wrong physical ideas about causality but in addition he did not yet understand some elementary mathematical notions about tensors (Pais, 1982, p. 224). 27
Einstein still had to understand that this freedom [to make “an arbitrary coordinate transformation”] expresses the fact that the choice of coordinates is a matter of convention without physical content (ibid., p. 222). 28
In 1979, Stachel started to study this question. Stachel (1979) presented a version of the standard
account, but by the following year it had become evident that this account was incorrect. At the 1980 Jena
meeting of the GRG Society, he presented a detailed analysis of the hole argument and its refutation by the
point coincidence argument; it circulated as a preprint, but was not published until 1989 (Stachel, 1989).
However, Torretti (1983 , Chapter 5.6) gives a detailed account of the hole argument based on
it;29
and Norton (1984), the first detailed analysis of Einstein’s 1913 Zurich
Notebook,30
also summarizes Stachel’s account.
, Chapter 5.6) gives a detailed account of the hole argument based on
it;29
and Norton (1984), the first detailed analysis of Einstein’s 1913 Zurich
Notebook,30
also summarizes Stachel’s account.
Stachel (1987) contains a historical-critical account of the hole argument, and Stachel (1986 ) uses the
fiber bundle formalism to generalize the argument to any geometric object field obeying generally-covariant
equations. These two talks helped to stimulate renewed interest in the meaning of diffeomorphism
invariance among relativists, especially those working on quantum gravity (see, e.g., Rovelli,
1991).
) uses the
fiber bundle formalism to generalize the argument to any geometric object field obeying generally-covariant
equations. These two talks helped to stimulate renewed interest in the meaning of diffeomorphism
invariance among relativists, especially those working on quantum gravity (see, e.g., Rovelli,
1991).
Earman and Norton’s presentations of the hole argument Earman and Norton
(1987 ); Earman (1989
); Earman (1989 ) provoked renewed discussion of absolute versus relational
theories of space-time among philosophers of science, a discussion that continues to this
day.31
Section 5 shows how several initially-different positions have converged on an approach that gives precise
meaning to Einstein’s vision of general relativity, and Section 6 reviews some physical topics related to the
hole argument.
) provoked renewed discussion of absolute versus relational
theories of space-time among philosophers of science, a discussion that continues to this
day.31
Section 5 shows how several initially-different positions have converged on an approach that gives precise
meaning to Einstein’s vision of general relativity, and Section 6 reviews some physical topics related to the
hole argument.
3.2 Einstein’s vision and fiber bundles
As we have seen already, Einstein often posed a problem, the solution to which required mathematical tools that went far beyond his current knowledge. Another example is the vision of the nature of general relativity that replaced his earlier faith in Mach’s principle (see Section 2.3). As we shall see, this new vision requires the theory of fiber bundles for its appropriate mathematical formulation.
When asked by a reporter to sum up the theory of relativity in a sentence, Einstein said, half jokingly:
Before my theory, people thought that if you removed all the matter from the universe, you would be left with empty space. My theory says that if you remove all the matter, space disappears, too! (Einstein, 1931). 32
In 1952, he developed the same idea at greater length:
On the basis of the general theory of relativity …space as opposed to “what fills space”
…has no separate existence. …If we imagine the gravitational field, i.e., the functions 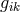 to be removed, there does not remain a space of the type (1) [Minkowski space-time],
but absolutely nothing, and also no “topological space”. …There is no such thing as an
empty space, i.e., a space without field. Space-time does not claim existence on its own,
but only as a structural quality of the field (Einstein, 1952
to be removed, there does not remain a space of the type (1) [Minkowski space-time],
but absolutely nothing, and also no “topological space”. …There is no such thing as an
empty space, i.e., a space without field. Space-time does not claim existence on its own,
but only as a structural quality of the field (Einstein, 1952 , p. 155).
, p. 155).
It is evident that this new approach to general relativity completely reverses his original Machian vision. Now the field is primary, and matter – like everything else – must be treated as an aspect of the field.
Einstein’s comment occurs in the course of a discussion of the age-old conflict between absolute33 and relational interpretations of space, which relativity theory metamorphosed into a conflict between interpretations of space-time.34
The quotation above stresses the role of the metric tensor, but elsewhere Einstein emphasizes the role of the affine connection, which he calls a displacement field:
It is the essential achievement of the general theory of relativity that it freed physics from
the necessity of introducing the “inertial system” (or inertial systems) …The development
…of the mathematical theories essential for the setting up of general relativity had the
result that at first the Riemannian metric was considered the fundamental concept on
which the general theory of relativity and thus the avoidance of the inertial system were
based. Later, however, Levi-Civita rightly pointed out that the element of the theory
that makes it possible to avoid the inertial system is rather the infinitesimal displacement
field 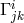 . The metric or the symmetric tensor field
. The metric or the symmetric tensor field 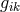 which defines it is only
indirectly connected with the avoidance of the inertial system in so far as it determines
a displacement field (Einstein, 1955, pp. 139 and 141).
which defines it is only
indirectly connected with the avoidance of the inertial system in so far as it determines
a displacement field (Einstein, 1955, pp. 139 and 141).
Einstein’s vision can be summed in the sentence: “Space-time does not claim existence on its own, but only as a structural quality of the field.” The two main elements of this vision are:
- If there is no field, there can be no space-time manifold.
- The spatio-temporal “structural qualities” of the field include the affine connection, which is actually of primary significance as compared to the metric tensor field.
Up until quite recently, the standard formulations of general relativity did not incorporate this vision. They start by postulating a four-dimensional differentiable manifold, which is described as a space-time before any metric tensor field is defined on it; and all other space-time structures, such as the Levi-Civita connection, are defined in terms of this one field.35 But the concepts of fiber bundles and sheaves enable a mathematical formulation of general relativity consistent with Einstein’s vision36 (see Section 4.3):
- If there is no total space for the fields, then there is no base manifold.
- The conceptual distinction between the roles of the metric and connection becomes evident: The metric lives on the vertical fibers of the total space; while the connection lives on the horizontal directions of the total space, connecting the fibers with each other.
Clearly, Einstein’s vision favors a non-absolutist view of
space-time.37
While no formalism can resolve a philosophical issue, the traditional approach that starts from a manifold
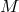 and defines various geometric object fields over it gives manifold substantivalists an initial advantage:
Opponents must explain away somehow the apparent priority of
and defines various geometric object fields over it gives manifold substantivalists an initial advantage:
Opponents must explain away somehow the apparent priority of 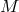 . The modern approach
starts from a principal fiber bundle
. The modern approach
starts from a principal fiber bundle 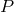 with total space
with total space  and structure group
and structure group 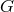 , and
defines
, and
defines 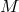 as the quotient
as the quotient 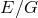 ; this gives non-substantivalists an initial advantage: The
whole bundle (pun intended), which includes some geometric object field, a connection and
a manifold, is there from the start; a manifold substantivalist must justify giving priority to
; this gives non-substantivalists an initial advantage: The
whole bundle (pun intended), which includes some geometric object field, a connection and
a manifold, is there from the start; a manifold substantivalist must justify giving priority to
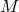 .
.